| Issue |
RAIRO-Theor. Inf. Appl.
Volume 40, Number 3, July-September 2006
Word Avoidability Complexity And Morphisms (WACAM)
|
|
|---|---|---|
| Page(s) | 443 - 458 | |
| DOI | https://doi.org/10.1051/ita:2006021 | |
| Published online | 18 October 2006 | |
On possible growths of arithmetical complexity
Sobolev Institute of Mathematics SB RAS, Koptyug av., 4, 630090
Novosibirsk, Russia;
frid@math.nsc.ru
The arithmetical complexity of infinite words, defined by Avgustinovich, Fon-Der-Flaass and the author in 2000, is the number of words of length n which occur in the arithmetical subsequences of the infinite word. This is one of the modifications of the classical function of subword complexity, which is equal to the number of factors of the infinite word of length n. In this paper, we show that the orders of growth of the arithmetical complexity can behave as many sub-polynomial functions. More precisely,
for each sequence u of subword complexity ƒu(n) and for each prime p ≥ 3 we build a Toeplitz word on the same alphabet whose arithmetical complexity is 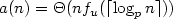 .
.
Mathematics Subject Classification: 68R15 / 68Q45
Key words: Arithmetical complexity / infinite word / subword complexity / Toeplitz word / bispecial words.
© EDP Sciences, 2006
Current usage metrics show cumulative count of Article Views (full-text article views including HTML views, PDF and ePub downloads, according to the available data) and Abstracts Views on Vision4Press platform.
Data correspond to usage on the plateform after 2015. The current usage metrics is available 48-96 hours after online publication and is updated daily on week days.
Initial download of the metrics may take a while.


